티스토리 뷰
Problem
Given an m x n integers matrix, return the length of the longest increasing path in matrix.
From each cell, you can either move in four directions: left, right, up, or down. You may not move diagonally or move outside the boundary (i.e., wrap-around is not allowed).
Example 1:
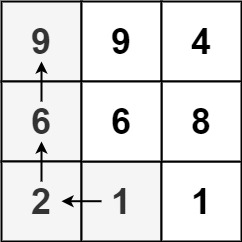
Input: matrix = [[9,9,4],[6,6,8],[2,1,1]]
Output: 4
Explanation: The longest increasing path is [1, 2, 6, 9].Example 2:
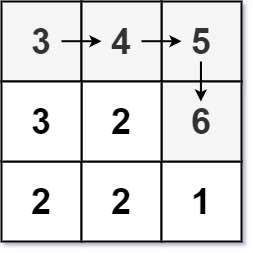
Input: matrix = [[3,4,5],[3,2,6],[2,2,1]]
Output: 4
Explanation: The longest increasing path is [3, 4, 5, 6]. Moving diagonally is not allowed.Example 3:
Input: matrix = [[1]]
Output: 1Constraints:
- m == matrix.length
- n == matrix[i].length
- 1 <= m, n <= 200
- 0 <= matrix[i][j] <= 2^31 - 1
Solution
m * n 행렬이 주어질 때 가장 긴 증가하는 경로를 구하는 문제입니다.
각 셀은 상하좌우로만 움직일 수 있고 대각선은 허용되지 않습니다.
DP와 DFS를 이용해 풀 수 있습니다.
public class Solution {
private static final int[][] DIRECTIONS = new int[][]{{1, 0}, {-1, 0}, {0, 1}, {0, -1}};
public int longestIncreasingPath(int[][] matrix) {
if (matrix == null || matrix.length == 0) {
return 0;
}
int result = 1;
int m = matrix.length;
int n = matrix[0].length;
int[][] dp = new int[m][n];
for (int i = 0; i < m; i++) {
for (int j = 0; j < n; j++) {
result = Math.max(result, dfs(matrix, dp, i, j));
}
}
return result;
}
private int dfs(int[][] matrix, int[][] dp, int i, int j) {
if (dp[i][j] > 0) {
return dp[i][j];
}
int max = 1;
for (int[] direction : DIRECTIONS) {
int x = i + direction[0];
int y = j + direction[1];
if (!isInBoundary(matrix, x, y) || matrix[x][y] <= matrix[i][j]) {
continue;
}
max = Math.max(max, 1 + dfs(matrix, dp, x, y));
}
return dp[i][j] = max;
}
private boolean isInBoundary(int[][] matrix, int x, int y) {
return x >= 0 && y >= 0 && x < matrix.length && y < matrix[0].length;
}
}DP 배열에는 누적된 경로의 길이를 저장합니다.
DFS 방식으로 각 셀마다 이동할 수 있는 경로를 탐색합니다.
이미 값이 0이 아닌 경우 누적된 길이가 저장되어있으므로 방문했다는 뜻입니다.
각 셀에서 상하좌우로 이동하면서 현재 값보다 더 큰 값을 가진 셀로 이동한 뒤 최댓값을 갱신하여 dp 배열에 할당하고 반환합니다.
이 과정을 반복하면서 결과도 계속 비교하여 최댓값을 구해야 합니다.
Test
package io.lcalmsky.leetcode.longest_increasing_path_in_a_matrix;
import static org.junit.jupiter.api.Assertions.assertAll;
import static org.junit.jupiter.api.Assertions.assertEquals;
import org.junit.jupiter.api.Test;
class SolutionTest {
@Test
void testAll() {
assertAll(
() -> test(new int[][]{{9, 9, 4}, {6, 6, 8}, {2, 1, 1}}, 4),
() -> test(new int[][]{{3, 4, 5}, {3, 2, 6}, {2, 2, 1}}, 4),
() -> test(new int[][]{{1}}, 1)
);
}
private void test(int[][] given, int expected) {
// when
Solution solution = new Solution();
int actual = solution.longestIncreasingPath(given);
// then
assertEquals(expected, actual);
}
}'Algorithm > LeetCode' 카테고리의 다른 글
| 47. Permutations II (0) | 2022.05.28 |
|---|---|
| 1302. Deepest Leaves Sum (0) | 2022.05.27 |
| 1091. Shortest Path in Binary Matrix (0) | 2022.05.23 |
| 743. Network Delay Time (0) | 2022.05.21 |
| 905. Sort Array By Parity (0) | 2022.05.20 |
댓글
공지사항
최근에 올라온 글
최근에 달린 댓글
- Total
- Today
- Yesterday
링크
TAG
- proto3
- 스프링 부트 애플리케이션
- @ManyToOne
- 헥사고날 아키텍처
- spring boot application
- 스프링 데이터 jpa
- intellij
- JSON
- Spring Data JPA
- Spring Boot
- r
- JPA
- Linux
- 함께 자라기
- spring boot app
- spring boot jwt
- 함께 자라기 후기
- Spring Boot JPA
- 스프링 부트 튜토리얼
- 스프링부트
- 스프링 부트 회원 가입
- 알고리즘
- QueryDSL
- Spring Boot Tutorial
- Java
- Jackson
- gRPC
- 클린 아키텍처
- leetcode
- 스프링 부트
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | |||
| 5 | 6 | 7 | 8 | 9 | 10 | 11 |
| 12 | 13 | 14 | 15 | 16 | 17 | 18 |
| 19 | 20 | 21 | 22 | 23 | 24 | 25 |
| 26 | 27 | 28 | 29 | 30 | 31 |
글 보관함
